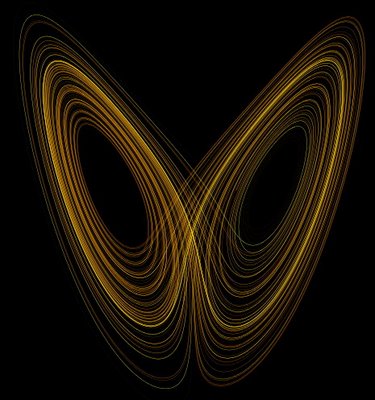
 A dynamical system has a state determined by a collection of real numbers, or more generally by a set of points in an appropriate state space. Small changes in the state of the system correspond to small changes in the numbers. The numbers are also the coordinates of a geometrical space—a manifold. The evolution rule of the dynamical system is a fixed rule that describes what future states follow from the current state. The rule is deterministic: for a given time interval only one future state follows from the current state.
A dynamical system has a state determined by a collection of real numbers, or more generally by a set of points in an appropriate state space. Small changes in the state of the system correspond to small changes in the numbers. The numbers are also the coordinates of a geometrical space—a manifold. The evolution rule of the dynamical system is a fixed rule that describes what future states follow from the current state. The rule is deterministic: for a given time interval only one future state follows from the current state.
20061223
Butter~Fly
The dynamical system concept is a mathematical formalization for any fixed "rule" which describes the time dependence of a point's position in its ambient space. The mathematical models used to describe the swinging of a clock pendulum, the flow of water in a pipe, or the number of fish each spring in a lake are examples of dynamical systems.
 A dynamical system has a state determined by a collection of real numbers, or more generally by a set of points in an appropriate state space. Small changes in the state of the system correspond to small changes in the numbers. The numbers are also the coordinates of a geometrical space—a manifold. The evolution rule of the dynamical system is a fixed rule that describes what future states follow from the current state. The rule is deterministic: for a given time interval only one future state follows from the current state.
A dynamical system has a state determined by a collection of real numbers, or more generally by a set of points in an appropriate state space. Small changes in the state of the system correspond to small changes in the numbers. The numbers are also the coordinates of a geometrical space—a manifold. The evolution rule of the dynamical system is a fixed rule that describes what future states follow from the current state. The rule is deterministic: for a given time interval only one future state follows from the current state.
 A dynamical system has a state determined by a collection of real numbers, or more generally by a set of points in an appropriate state space. Small changes in the state of the system correspond to small changes in the numbers. The numbers are also the coordinates of a geometrical space—a manifold. The evolution rule of the dynamical system is a fixed rule that describes what future states follow from the current state. The rule is deterministic: for a given time interval only one future state follows from the current state.
A dynamical system has a state determined by a collection of real numbers, or more generally by a set of points in an appropriate state space. Small changes in the state of the system correspond to small changes in the numbers. The numbers are also the coordinates of a geometrical space—a manifold. The evolution rule of the dynamical system is a fixed rule that describes what future states follow from the current state. The rule is deterministic: for a given time interval only one future state follows from the current state.
訂閱:
張貼留言 (Atom)

沒有留言:
張貼留言